Pascal Pirlot, Ph.D. Candidate 36th cycle, University of Trento, DICAM
In the vast majority of unmodified catchments around the world, rivers exiting steep and narrowly confined mountainous regions access a wider, flatter valley. There, the river morphology is no longer determined mainly by geological constraints, but rather by the dynamic equilibrium between water flow, alluvial sediment and vegetation. The widening of the floodplain is related to the transverse channel migration caused by the flow concentration eroding one channel bank while depositing sediment on the other. The tendency to split into anabranches is related to the flow efficiency (i.e. the sediment transport capacity per unit stream power) and the ability of a channel to form central bars. At a bifurcation, a wide and shallow channel, such as found in wide floodplains, splits the water and sediment fluxes unevenly along the bifurcates. This uneven flux partition is promoted by the omnipresent asymmetric conditions: channel curvature, migration of bars and asymmetric backwater effects. Given this intrinsic unbalanced flux partition at each bifurcation, the heterogeneous alluvial cohesion and vegetation distribution, the multi-thread river type presents a complex morphology. This complexity retroactively entertains a very high diversity in channel width and slope, flow velocity and sediment grain size, but also in water temperature, illuminance, nutrient concentrations, which contributes to flow retention and habitat richness and resilience.
My work aims at deepening existing contributions about nodal bifurcations where banks are easily erodible, as typically the case of braided rivers, and exploring the connection between the bifurcation dynamics and the morphological complexity of multi-thread patterns.
The structure of my work divides into tackling the following tasks:
- reproduce and develop a semi-analytical model that describes bifurcation geometry at equilibrium under bankfull discharge condition and compare the outputs to an existing data set about channel loops;
- develop a methodology to define bifurcation units and measure their morphological features based on the analysis of digital terrain models;
- determine expected geometrical features at the reach-scale;
- reconstruct the evolution of two bifurcations during a series of flood events on the Tagliamento River and withdraw information for boundary conditions from it.
For now, only the first two steps have been achieved. The bankfull uniform flow bifurcation model is improved by considering bank erosion and coupling the channel discharge to its slope and width using a regime formula:
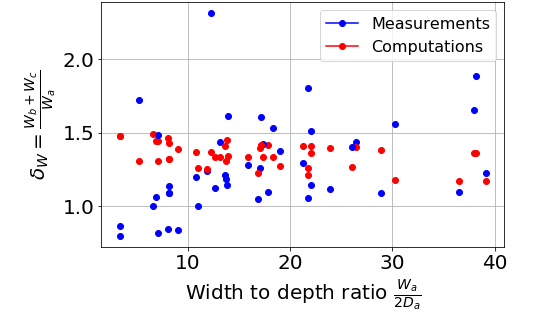
Weq = αQβg −β/2 ρβSβd−5β/2 +150
where Weq is the channel width, Q the water discharge in [m3.s−1], g the earth’s acceleration in [m.s−2], ρ the water density in [kg.m−3], d50 the median grain diameter in [m], S the channel slope in [m/m] and α and β two chosen scalars. By calibrating α in each river, a flow regime is defined for the flow conditions in which the satellite photo is taken and is applied to evaluate the width of the bifurcates. I defined δW = (Wb+Wc)/Wa as the “channel enlargement”. Figure 2 compares the observed enlargement to the computed one. The model is able to capture the channel enlargement qualitatively (90% of the bifurcations have both δW > 1) but there is no clear trend to notice yet, I will expand the data set to braided and anastomosing rivers.
Figure 1:

Figure 2: